
- 2.1: Taking Measurements
- Chemists measure the properties of matter and express these measurements as quantities. A quantity is an amount of something and consists of a number and a unit. The number tells us how many (or how much), and the unit tells us what the scale of measurement is. For example, when a distance is reported as '5 kilometers,' we know that the quantity has been expressed in units of kilometers and that the number of kilometers is 5.
- 2.2: Scientific Notation - Writing Large and Small Numbers
- Chemists often work with numbers that are exceedingly large or small. For example, entering the mass in grams of a hydrogen atom into a calculator requires a display with at least 24 decimal places. A system called scientific notation avoids much of the tedium and awkwardness of manipulating numbers with large or small magnitudes.
- 2.3: Significant Figures - Writing Numbers to Reflect Precision
- Uncertainty exists in all measurements. The degree of uncertainty is affected in part by the quality of the measuring tool. Significant figures give an indication of the certainty of a measurement. Rules allow decisions to be made about how many digits to use in any given situation.
- 2.4: Significant Figures in Calculations
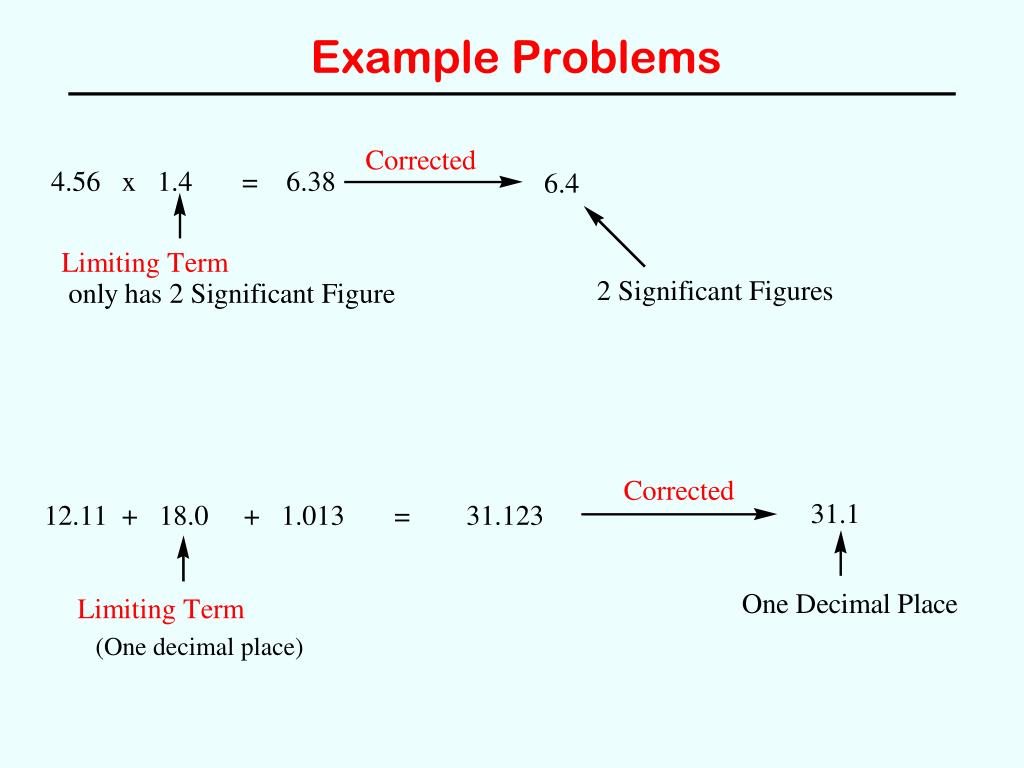
- To round a number, first decide how many significant figures the number should have. Once you know that, round to that many digits, starting from the left. If the number immediately to the right of the last significant digit is less than 5, it is dropped and the value of the last significant digit remains the same. If the number immediately to the right of the last significant digit is greater than or equal to 5, the last significant digit is increased by 1.
- 2.5: The Basic Units of Measurement
- Metric prefixes derive from Latin or Greek terms. The prefixes are used to make the units manageable. The SI system is based on multiples of ten. There are seven basic units in the SI system. Five of these units are commonly used in chemistry.
- 2.6: Problem Solving and Unit Conversions
- During your studies of chemistry (and physics also), you will note that mathematical equations are used in a number of different applications. Many of these equations have a number of different variables with which you will need to work. You should also note that these equations will often require you to use measurements with their units. Algebra skills become very important here!
- 2.7: Solving Multistep Conversion Problems
- Sometimes you will have to perform more than one conversion to obtain the desired unit.
- 2.8: Units Raised to a Power
- Conversion factors for area and volume can also be produced by the dimensional analysis method. Just remember that if a quantity is raised to a power of 10 both the number and the unit must be raised to the same power of 10.
- 2.9: Density
- Density is a physical property found by dividing the mass of an object by its volume. Regardless of the sample size, density is always constant.
- 2.E: Homework Chapter 2
- 2.E: Homework Chapter 2 Answers
- 2.6: Problem Solving and Unit Conversions During your studies of chemistry (and physics also), you will note that mathematical equations are used in a number of different applications. Many of these equations have a number of different variables with which you will need to work.
- Everyday world.The materials are organized by chapter and lesson, with one Word Problem Practice worksheet for every lesson in Glencoe Math Connects, Course 2. Always keep your workbook handy.

This section of the competition consists of 30 problems. You will have 40 minutes to complete all the problems. You are not allowed to use calculators, books or other aids during this round. If you are wearing a calculator wrist watch, please give it to your proctor now. Calculations may be done on scratch paper. All answers must be complete. Problem Solving. Represent Data Learning Objective Solve problem situations using the strategy make a graph. Language Objective Children present to a partner, using at least one example of how showing information in a graph can help you solve problems. Materials MathBoard F C R Focus: Common Core State Standards.
Josie is a kindergarten teacher and wants to make felt toys for her students. These felt toys will be cylinders, meant to replicate cans. She wants to put the felt toys in the new play kitchen in her classroom. To find the amount of felt she needs, Josie will need to calculate the surface area of the cans she plans to make. This chapter will explain how to find surface area of different objects, including cylinders.
Learning Outcomes
By the end of this section, you will be able to:
- Find volume and surface area of rectangular solids
- Find volume and surface area of spheres
- Find volume and surface area of cylinders
- Find volume of cones
Before you get started in this module, try a few practice problems and review prior concepts.
Math Problem Solving Questions
readiness quiz
- Evaluate [latex]{x}^{3}[/latex] when [latex]x=5[/latex].If you missed this problem, review [link].
- Evaluate [latex]{2}^{x}[/latex] when [latex]x=5[/latex].If you missed this problem, review [link].
- Find the area of a circle with radius [latex]frac{7}{2}[/latex].If you missed this problem, review [link].

Chapter Round Materialmath Problem Solving
In this section, we will finish our study of geometry applications. We find the volume and surface area of some three-dimensional figures. Since we will be solving applications, we will once again show our Problem-Solving Strategy for Geometry Applications.
Mount and blade warband marry claimant. Marrying claimant Playing Floris, trying to marry Lady Isolla of Suno, as I made her the ruler of Swadia. I have the more renown of our whole faction, 0 controversy, 12 right to rule (growing to the point she's already ♥♥♥♥♥♥ about it). The option for marrying the claimant will not show up unless the player has completed the quest and defeated the original faction. Good relations with ruler. High renown, honour and right to rule The ruler will refuse to marry the player if he/she does not have enough honor. Another new option in Mount & Blade: Warband is to woo a lady with poetry and marry her. You can do it in secret or you can get her father's permission (sometimes brother). It takes several visits to worm your way into a bedchamber and it's not a good idea to rush. You can learn poems in taverns and there seems to be a limit of five in total.
Chapter Round Materialmath Problem Solving Problem
5othird grade james tes. Problem Solving Strategy for Geometry Applications
- 2.1: Taking Measurements
- Chemists measure the properties of matter and express these measurements as quantities. A quantity is an amount of something and consists of a number and a unit. The number tells us how many (or how much), and the unit tells us what the scale of measurement is. For example, when a distance is reported as '5 kilometers,' we know that the quantity has been expressed in units of kilometers and that the number of kilometers is 5.
- 2.2: Scientific Notation - Writing Large and Small Numbers
- Chemists often work with numbers that are exceedingly large or small. For example, entering the mass in grams of a hydrogen atom into a calculator requires a display with at least 24 decimal places. A system called scientific notation avoids much of the tedium and awkwardness of manipulating numbers with large or small magnitudes.
- 2.3: Significant Figures - Writing Numbers to Reflect Precision
- Uncertainty exists in all measurements. The degree of uncertainty is affected in part by the quality of the measuring tool. Significant figures give an indication of the certainty of a measurement. Rules allow decisions to be made about how many digits to use in any given situation.
- 2.4: Significant Figures in Calculations
- To round a number, first decide how many significant figures the number should have. Once you know that, round to that many digits, starting from the left. If the number immediately to the right of the last significant digit is less than 5, it is dropped and the value of the last significant digit remains the same. If the number immediately to the right of the last significant digit is greater than or equal to 5, the last significant digit is increased by 1.
- 2.5: The Basic Units of Measurement
- Metric prefixes derive from Latin or Greek terms. The prefixes are used to make the units manageable. The SI system is based on multiples of ten. There are seven basic units in the SI system. Five of these units are commonly used in chemistry.
- 2.6: Problem Solving and Unit Conversions
- During your studies of chemistry (and physics also), you will note that mathematical equations are used in a number of different applications. Many of these equations have a number of different variables with which you will need to work. You should also note that these equations will often require you to use measurements with their units. Algebra skills become very important here!
- 2.7: Solving Multistep Conversion Problems
- Sometimes you will have to perform more than one conversion to obtain the desired unit.
- 2.8: Units Raised to a Power
- Conversion factors for area and volume can also be produced by the dimensional analysis method. Just remember that if a quantity is raised to a power of 10 both the number and the unit must be raised to the same power of 10.
- 2.9: Density
- Density is a physical property found by dividing the mass of an object by its volume. Regardless of the sample size, density is always constant.
- 2.E: Homework Chapter 2
- 2.E: Homework Chapter 2 Answers
- 2.6: Problem Solving and Unit Conversions During your studies of chemistry (and physics also), you will note that mathematical equations are used in a number of different applications. Many of these equations have a number of different variables with which you will need to work.
- Everyday world.The materials are organized by chapter and lesson, with one Word Problem Practice worksheet for every lesson in Glencoe Math Connects, Course 2. Always keep your workbook handy.
This section of the competition consists of 30 problems. You will have 40 minutes to complete all the problems. You are not allowed to use calculators, books or other aids during this round. If you are wearing a calculator wrist watch, please give it to your proctor now. Calculations may be done on scratch paper. All answers must be complete. Problem Solving. Represent Data Learning Objective Solve problem situations using the strategy make a graph. Language Objective Children present to a partner, using at least one example of how showing information in a graph can help you solve problems. Materials MathBoard F C R Focus: Common Core State Standards.
Josie is a kindergarten teacher and wants to make felt toys for her students. These felt toys will be cylinders, meant to replicate cans. She wants to put the felt toys in the new play kitchen in her classroom. To find the amount of felt she needs, Josie will need to calculate the surface area of the cans she plans to make. This chapter will explain how to find surface area of different objects, including cylinders.
Learning Outcomes
By the end of this section, you will be able to:
- Find volume and surface area of rectangular solids
- Find volume and surface area of spheres
- Find volume and surface area of cylinders
- Find volume of cones
Before you get started in this module, try a few practice problems and review prior concepts.
Math Problem Solving Questions
readiness quiz
- Evaluate [latex]{x}^{3}[/latex] when [latex]x=5[/latex].If you missed this problem, review [link].
- Evaluate [latex]{2}^{x}[/latex] when [latex]x=5[/latex].If you missed this problem, review [link].
- Find the area of a circle with radius [latex]frac{7}{2}[/latex].If you missed this problem, review [link].
Chapter Round Materialmath Problem Solving
In this section, we will finish our study of geometry applications. We find the volume and surface area of some three-dimensional figures. Since we will be solving applications, we will once again show our Problem-Solving Strategy for Geometry Applications.
Mount and blade warband marry claimant. Marrying claimant Playing Floris, trying to marry Lady Isolla of Suno, as I made her the ruler of Swadia. I have the more renown of our whole faction, 0 controversy, 12 right to rule (growing to the point she's already ♥♥♥♥♥♥ about it). The option for marrying the claimant will not show up unless the player has completed the quest and defeated the original faction. Good relations with ruler. High renown, honour and right to rule The ruler will refuse to marry the player if he/she does not have enough honor. Another new option in Mount & Blade: Warband is to woo a lady with poetry and marry her. You can do it in secret or you can get her father's permission (sometimes brother). It takes several visits to worm your way into a bedchamber and it's not a good idea to rush. You can learn poems in taverns and there seems to be a limit of five in total.
Chapter Round Materialmath Problem Solving Problem
5othird grade james tes. Problem Solving Strategy for Geometry Applications
Math Solving Calculator
- Read the problem and make sure you understand all the words and ideas. Draw the figure and label it with the given information.
- Identify what you are looking for.
- Name what you are looking for. Choose a variable to represent that quantity.
- Translate into an equation by writing the appropriate formula or model for the situation. Substitute in the given information.
- Solve the equation using good algebra techniques.
- Check the answer in the problem and make sure it makes sense.
- Answer the question with a complete sentence.
